Question

A jet airliner moving initially at 399 mph

(with respect to the ground) to the east moves
into a region where the wind is blowing at
552 mph in a direction 72◦
north of east.
What is the new speed of the aircraft with
respect to the ground?
Answer in units of mph.

Answer 1

The new speed of the aircraft is equal to 774.61 mph north of east.

Why?

To calculate the new speed, we first need to know the horizontal component of the wind's speed (moving to the east) since the jet is moving to the east.

We can use the following formulas to solve the problem:

`WindSpeed_(x)=WindSpeed*Cos(\alpha )\\\\HorizontalSpeed_(x)=InitialSpeed+WindSpeed_(x)`

We are given the following information:

`InitialSpeed=399mph\\WindSpeed=552mph\\\alpha =72\°`

So, substituting and calculating we have:

`WindSpeed_(x)=552mph*Cos(72\° )=170.58mph\\\\HorizontalSpeed_(x)=399mph+170.58mph=569.58mph`

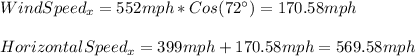
For the vertical speed, we have:

`WindSpeed_(y)=552mph*Sin(72\° )=524.98mph`

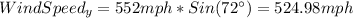
Now, to calculate the airplane speed , we can use the following equation (Pytagorean Theorem):

`FinalSpeed=\sqrt{HorizontalSpeed^(2) +VerticalSpeed^(2)}\\\\FinalSpeed=\sqrt{569.58mph^(2) +524.98mph^(2)}\\\\FinalSpeed=774.61mph`

Hence, we have that the new speed is equal to 774.61 mph north of east.

Have a nice day!