Question

Jenna has bought a new hybrid car. Each week for a period of seven weeks, she has noted the fuel efficiency (in miles per gallon) of her car.

The results are shown below. 45 44 43 44 45 44 43 6. Calculate the standard deviation of these results to the nearest hundredth. Be sure to show your work.

Answer 1

Answer: 0.82 miles per gallon

Explanation:

Given : Jenna has bought a new hybrid car. Each week for a period of seven weeks, she has noted the fuel efficiency (x) (in miles per gallon) of her car.

The results are :45 44 43 44 45 44 43

The formula to find the standard deviation is given by :-

`\sigma=\sqrt{\frac{\sum_(i=1)^n(x_i-\overline{x})^2}{n-1}}`

First we find mean of the given data ,

`\overline{x}=(\sum^n_(i=1)x_i)/(n)\\\\=(45+44+43+44+45+44+43)/(7)=(308)/(7)=44`

Now,

`{\sum_(i=1)^n(x_i-\overline{x})^2=(1)^2+(0)^2+(-1)^2+(0)^2+(1)^2+(0)^2+(-1)^2`

`=1+1+1+1=4`

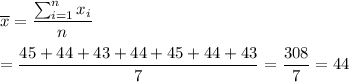
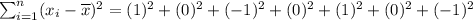
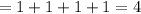
Now, standard deviation of the above results will be :-

`\sigma=\sqrt{(4)/(7-1)}\\\\=\sqrt{(4)/(6)}\\\\=(2)/(√(3))=0.816496580928\approx0.82`

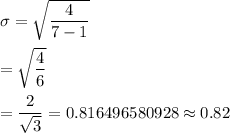
Hence, the standard deviation of these results = 0.82 miles per gallon