Question

18 PTS! From the equation below, identify the slope and a point on the line. y−3=−2(x+1)

Answer 1

Answer: slope: − 2

y-intercept: ( 0 , 1 )

x y 0 1 1 − 1

Step-by-step explanation: Graph the line using the slope and y-intercept, or two points.

Answer 2

The slope of the given equation is -2 and a point on the given line is (-1, 3)

SOLUTION:

Given, linear equation in two variables is y – 3 = -2(x + 1) ----- eqn (1)

We need to find the slope of the given equation and a point through which the given line passes.

Given equation is in the form of point slope form .i.e
`\mathrm{y}-\mathrm{y}_(1)=\mathrm{m}\left(\mathrm{x}-\mathrm{x}_(1)\right)` --- eqn 2

where,"m" is the slope of the line

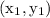`\left(\mathrm{x}_(1), \mathrm{y}_(1)\right)` is point on that line.

Now, by comparing (1) and (2)

m = -2

`x_(1)=-1`

`y_(1)=3`

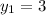
so, the slope of the given equation is -2 and a point on the given line is (-1, 3)