Question

Of all the trees planted by a landscaping firm, 45% survive. What is the probability that 13 or more of the 15 trees they just planted will survive

Answer 1

The probability for 13 or more of the 15 trees they just planted will survive is 0.00110702414

SOLUTION:

Given,

The total number of plants is, n=15

The chance of survival of trees is, p=45%.

Probability of getting success p(success) =
`\frac{n o \text { of favourable outcomes }}{\text {total possible outcomes}}`

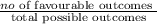
P(success) =
`(45)/(100)`

P(success) = 0.45

Binomial distribution formula is given as

`\mathrm{P}(\mathrm{X}=\mathrm{x})=\mathrm{n}_{\mathrm{C} \mathrm{x}}(\mathrm{p})^{\mathrm{x}} \cdot(1-\mathrm{p})^{\mathrm{n}-\mathrm{x}}`

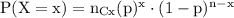
In our case, x is greater than or equal to 13, i.e. x
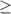`\geq` 13

The probability for 13 or more of the 15 trees they just planted will survive is given by

`\mathrm{P}(\mathrm{X} \geq 13)=\mathrm{P}(\mathrm{X}=13)+\mathrm{P}(\mathrm{X}=14)+\mathrm{P}(\mathrm{X}=15)`

`\mathrm{P}(\mathrm{X} \geq 13)=\left(15 \mathrm{C}_(13) *(0.45)^(13) *(1-0.45)^(15-13)\right.` +
`\left(^(15) \mathrm{C}_(14) *(0.45)^(14) *(1-0.45)^(15-14)\right.` +
`\left(15 \mathrm{C}_(15) *(0.45)^(15) *(1-0.45)^(15-15)\right.`

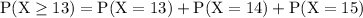
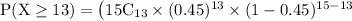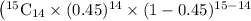on simplification we get

`=(15 * 7) *(0.45)^(13) *(0.55)^(2)+15 *(0.45)^(14) *(0.55)^(1)+1 *(0.45)^(15) * 1`

`\begin{array}{c}{=(105 * 0.00003102863 * 0.3025)+(15 * 0.00001396288 * 0.55)+} \\ {0.00000628329}\end{array}`

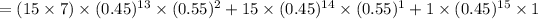
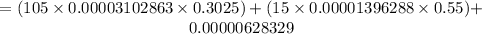
=0.00098554703 + 0.0001151938 + 0.00000628329

= 0.00110702414

Hence, the probability for 13 or more of the 15 trees they just planted will survive is 0.00110702414