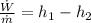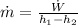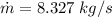Question

Consider a Rankine cycle, where steam enters the turbine @ 5 Mpa, 500 °C and exits at 12.35 kPa. The cycle produce 10,000 KW of electricity. Determine the cycle efficiency and the steam amount needed. (in)

Answer 1

cycle efficiency: 0.11

steam amount needed: 8.327 kg/s

Step-by-step explanation:

Points 1,2,3 and 4 are depicted in first figure attached. h means enthalpy and s means entropy .

Assumption
`s_2 = s_1`

Interpolation to get
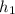`h_1` (see second figure)

p (MPa) h (kJ/kg)

4 3445.3

5
`h_1`

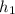
6 3422.2

(3445.3 - 3422.2)/(4 - 6) = (3445.3 -
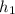`h_1`)/(4 - 5)

`h_1 = 3433.75 \; kJ/kg`

In a similar way
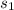`s_1` is computed as 6.9852 kJ/(kg K)

At 12.35 kPa, i. e., 0.1235 bar (see third figure):
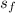`s_f` = 0.6922;
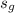`s_g` = 8.093;
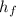`h_f` = 205.82 ;
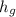`h_g` = 2590.575 (again from interpolation, units omitted)

Quality (x) =
`(s_2 - s_f)/(s_g - s_f) = 0.85`

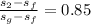
Enthalpy =
`h_2 = (h_g - h_f)*x + h_f = 2232.86`

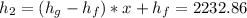
In a similar way
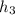`h_3` is computed as 205.83 kJ/kg.

Point 4 is computed as saturated liquid at 5 MPa; then,
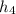`h_4` = 1154.2 kJ/kg (see fourth figure).

Efficiency is calculated as

`\eta = 1 - (h_2 - h_3)/(h_1 - h_4)`

`\eta = 1 - (2232.86 - 205.83)/(3433.75 - 1154.2)`

`\eta = 0.11`

Energy balance at turbine gives

`\frac{\dot{W}}{\dot{m}} = h_1 - h_2`

`\dot{m} = \frac{\dot{W}}{h_1 - h_2}`

`\dot{m} = (10,000 \; KW)/(3433.75 \; kJ/kg - 2232.86 \; kJ/kg)`

`\dot{m} = 8.327 \; kg/s`