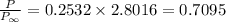Answer:
At the point of minimum pressure,
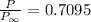
Solution:
As per the question;
Critical Mach no. for the air foil,
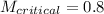
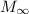 = 0.8
= 0.8
Now, for the value of the minimum pressure point:
Pressure of the air foil is critical,
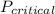 when
when
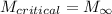
Now,
 (1)
(1)
When M = 1:
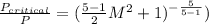
 (2)
(2)
When
 :
:
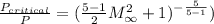
 (3)
(3)
Now, from the eqns (1), (2) and (3):