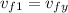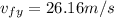Answer:
(a) t = 2.67 s
(b) x= 293.7 m
(c)
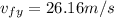
Step-by-step explanation:
Conceptual analysis:
Projectile movement is semi-parabolic,then, We aply the following formulas:
y = y₀+ v₀₁t + (1/2)*g*t² Formula 1
x= v₀ₓ * t Formula 2
vf₁= v₀₁+g*t Formula 3
Where
x: horizontal distance (m)
v₀ₓ : initial speed in x of the projectile (m/s)
y: final height of the projectile (m)
y₀ : initial height of the projectile (m)
v₀₁: initial speed in y of the projectile (m/s)
vf₁: final speed in y of the projectile (m/s)
g : acceleration due to gravity ( m/s²)
t : time (s)
Known data
y₀= -35 m
v₀ₓ = 110 m/s
v₀₁ = 0
g = 9.8 m/s²
t = time (s)
Problem development
We replace data in formula (1) to calculate the time that the projectile remains in the air, that is, when y = 0.
Because the projectile moves down the sign of the equation is positive in the term (1/2)*g*t²:
a)y = y₀+ v₀₁t + (1/2)*(g)*(t)²
0 = -35 +0+ (1/2) *(9.8) (t)²
35= 4.9 *(t)²
t² = 35/4.9
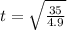
t = 2.67 s
b) We apply the formula 2 to calculate horizontal distance from the firing point does it strike the ground:
x= 110 m/s * 2.67 s
x= 293.7 m
c) Magnitude of the vertical component of its velocity as it strikes the ground
We replace data in Formula 3
vf₁= v₀₁+g*t
vf₁= 0+9.8*2.67 = 26.16 m/s