Question

A volume of rock has a mass roughly three times a similar volume of ice. Suppose one world is made of ice whereas another world with the same radius is made of rock. If g is the acceleration of gravity on the surface of the ice world, what is the approximate acceleration of gravity on the rock world?

Answer 1

Acceleration on the rock world will be 3 times the acceleration on the ice world

Step-by-step explanation:

Let the mass of the ice is
`m_i`

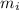
So mass of rock
`m_r=3m_i`

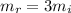
It is given that both have same radius, let radius is r

We know that acceleration due to gravity is given by
`g=(GM)/(R^2)`

So acceleration due to ice world
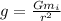`g=(Gm_i)/(r^2)` ----eqn 1

Acceleration of gravity on rock world
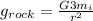`g_(rock)=(G3m_i)/(r^2)`-----eqn 2

Now dividing eqn 1 by eqn 2

`(g)/(g_(rock))=(1)/(3)`

`g_(rock)=3g`

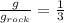
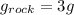
So acceleration on the rock world will be 3 times the acceleration on the ice world