Question

The floor of a railroad flatcar is loaded with loose crates having a coefficient of static friction of 0.480 with the floor. If the train is initially moving at a speed of 61.0 km/h, in how short a distance can the train be stopped at constant acceleration without causing the crates to slide over the floor?

Answer 1

Answer:30.50 m

Step-by-step explanation:

Given

coefficient of friction between railroad and crates
`\mu =0.48`

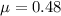
Train initial velocity (u)
`=61 kmph \approx 16.94 m/s`

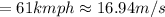
To get the shortest distance brakes applied should be order of friction force between crates and railroad floor

`a=\mu g=0.48* 9.8=4.704 m/s^2`

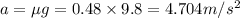
using
`v^2-u^2=2as`

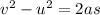
where v=final velocity

u=initial velocity

a=acceleration or deceleration

s=distance

here v=0 , u=16.94 m/s

`0-(16.94)^2=2* (-4.704)* s`

`s=((16.94)^2)/(2* 4.704)`

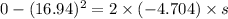
s=30.502 m