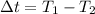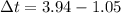Question

One strategy in a snowball fight is to throw a snowball at a high angle over level ground. While your opponent is watching the first one, a second snowball is thrown at a low angle timed to arrive before or at the same time as the first one. Assume both snowballs are thrown with a speed of 20.0 m/s. The first one is thrown at an angle of 75.0° with respect to the horizontal. (a) at what angle should the second snowball be thrown to arrive at the same point as the first? (b) How many seconds later should the second snowball be thrown after the first to arrive at the same time?

Answer 1

Part a)

`\theta_2 = 15 degree`

Part b)

`\Delta t = 2.88 s`

Step-by-step explanation:

Part a)

In order to have same range for same initial speed we can say

`R_1 = R_2`

`(v^2 sin2\theta_1)/(g) = (v^2 sin2\theta_2)/(g)`

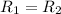
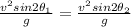
so after comparing above we will have

`\theta_1 = 90 - \theta`

so we have

`75 = 90 - \theta_2`

`\theta_2 = 15 degree`

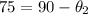
Part b)

Time of flight for the first ball is given as

`T_1 = (2vsin\theta)/(g)`

`T_1 = (2(20)sin75)/(9.81)`

`T_1 = 3.94 s`

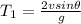
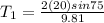
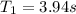
Now for other angle of projection time is given as

`T_2 = (2(20)sin15)/(9.81)`

`T_2 = 1.05 s`

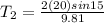
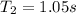
So here the time lag between two is given as

`\Delta t = T_1 - T_2`

`\Delta t = 3.94 - 1.05`

`\Delta t = 2.88 s`