Answer:
The expected value for your profit is -$0.10
Explanation:
The expected value of a discrete variable is calculated as:

Where,
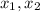 and
and
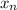 are the values that the variable can take and
are the values that the variable can take and
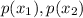 and
and
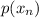 are their respective probabilities.
are their respective probabilities.
So, the expected value of your income is:
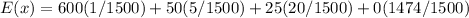
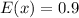
Because, you can win $600 with a probability of 1/1500, $50 with a probability of 5/1500, $25 with a probability of 20/1500 or $0 with a probability of 1474/1500.
Then, if you buy a ticket for $1, the expected value for your profit is:
Expected Value = Expected Income - Cost
Expected Value = $0.9 - $1
Expected Value = -$0.1