Question

A jet airliner moving initially at 858 mph (with respect to the ground) to the east moves into a region where the wind is blowing at 777 mph in a direction 38◦ north of east. What is the new speed of the aircraft with respect to the ground?

Answer 1

1546.2 mph

Step-by-step explanation:

The new speed of the aircraft will be the magnitude of the resultant velocity due to the velocity of the aircraft + the velocity of the wind.

The components of the initial velocity of the jet are:

`v_x = 858 mph\\v_y = 0`

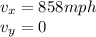
where we took east as positive x-direction and north as positive y-direction.

The components of the wind's velocity are

`v'_x = (777)(cos 38^(\circ))=612.3 mph\\v'_y = (777)(sin 38^(\circ))=478.4 mph`

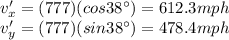
So the components of the resultant velocity are

`V_x = v_x + v'_x = 858+612.3=1470.3 mph\\V_y = v_y+v'_y = 0+478.4 = 478.4 mph`

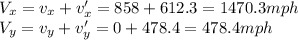
And so, the new speed of the plane respect to the ground is

`V=√((1470.3)^2+(478.4)^2)=1546.2 mph`