Answer:
Explanation:
(a) What is the mean fish weight of your catch of 6? Round your answer to 1 decimal place. =Total weight/no of fish in sample
_
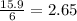 _____ pounds
_____ pounds
(b) If 6 bass are randomly selected from Clear Lake, find the probability that the mean weight is greater than the mean of those you caught. Round your answer to 4 decimal places.
___P(mean >2.65)=
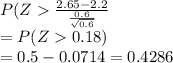 _____
_____
Sample mean follows a normal distribution with mean = 2.2 and std error = 0.6/sqrt 6
(c) Which statement best describes your situation?
_____ This is not particularly unusual because the mean weight of your fish is only 0.5 pounds above the population average.