Question

The Economic Policy Institute periodically issues reports on wages of entry level workers. The Institute reported that entry level wages for male college graduates were $21.68 per hour and for female college graduates were $18.80 per hour in 2011 (Economic Policy Institute website, March 30, 2012). Assume the standard deviation for male graduates is $2.30, and for female graduates it is $2.05 What is the probability that a sample of 50 male graduates will provide a sample mean within $.50 of the population mean, $21.68?

What is the probability that a sample of 50 female graduates will provide a sample mean within $.50 of the population mean, $18.80?

What is the probability that a sample of 120 female graduates will provide a sample mean more than $.30 below the population mean?

Answer 1

The Economic Policy Institute periodically issues reports on wages of entry level workers. The Institute reported that entry level wages for male college graduates were $21.68 per hour and for female college graduates were $18.8

Explanation:

Answer 2

87.64% probability that a sample of 50 male graduates will provide a sample mean within $.50 of the population mean, $21.68.

91.46% probability that a sample of 50 female graduates will provide a sample mean within $.50 of the population mean, $18.80.

5.48% probability that a sample of 120 female graduates will provide a sample mean more than $.30 below the population mean.

Explanation:

The Central Limit Theorem estabilishes that, for a random variable X, with mean
`\mu` and standard deviation
`\sigma`, a large sample size can be approximated to a normal distribution with mean
`\mu` and standard deviation
`(\sigma)/(√(n))`.

Problems of normally distributed samples can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

So, lets see each question:

What is the probability that a sample of 50 male graduates will provide a sample mean within $.50 of the population mean, $21.68?

The Institute reported that entry level wages for male college graduates were $21.68 per hour, with a standard deviation of 2.30.

So
`\mu = 21.68, \sigma = 2.30, n = 50, s = (2.30)/(√(50)) = 0.3253`

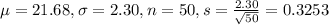
This probability is the pvalue of Z when
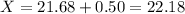`X = 21.68 + 0.50 = 22.18` subtracted by the pvalue of Z when
`X = 21.68 - 0.50 = 21.18`

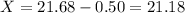
X = 22.18

`Z = (X - \mu)/(s)`

`Z = (22.18 - 21.68)/(0.3253)`

`Z = 1.54`

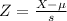
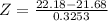
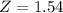
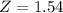`Z = 1.54` has a pvalue of 0.9382.

X = 21.18

`Z = (X - \mu)/(s)`

`Z = (21.18 - 21.68)/(0.3253)`

`Z = -1.54`

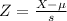
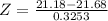
`Z = -1.54` has a pvalue of 0.0618.

There is a 0.9382 - 0.0618 = 0.8764 = 87.64% probability that a sample of 50 male graduates will provide a sample mean within $.50 of the population mean, $21.68.

What is the probability that a sample of 50 female graduates will provide a sample mean within $.50 of the population mean, $18.80?

`\mu = 18.80, \sigma = 2.05, n = 50, s = (2.05)/(√(50)) = 0.29`

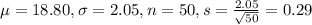
This probability is the pvalue of
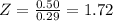`Z = (0.50)/(0.29) = 1.72` subtracted by the pvalue of
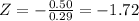`Z = -(0.50)/(0.29) = -1.72`, following the same logic as the question above.

There is a 0.9573 - 0.0427 = 0.9146 = 91.46% probability that a sample of 50 female graduates will provide a sample mean within $.50 of the population mean, $18.80.

What is the probability that a sample of 120 female graduates will provide a sample mean more than $.30 below the population mean?

`\mu = 18.80, \sigma = 2.05, n = 120, s = (2.05)/(√(120)) = 0.1871`

This is the pvalue of Z when
`X = 18.80 - 0.30 = 18.50`

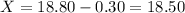
So

`Z = (X - \mu)/(\sigma)`

`Z = (18.50 - 18.80)/(0.1871)`

`Z = - 1.6`

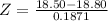
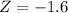
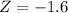`Z = - 1.6` has a pvalue of 0.0548.

So there is a 5.48% probability that a sample of 120 female graduates will provide a sample mean more than $.30 below the population mean.