Question

Which statement correctly describes the relationship between the graph of f(x) and g(x)=f(x+2) ?

The graph of g(x) is the graph of ​f(x)​ translated 2 units down.

The graph of g(x) is the graph of ​f(x)​ translated 2 units right.

The graph of g(x) is the graph of ​f(x)​ translated 2 units up.

The graph of g(x) is the graph of ​f(x)​ translated 2 units left.

Answer 1

Option D.

Explanation:

The transformation of a function is defined as

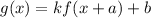`g(x)=kf(x+a)+b` .... (1)

Where, k is stretch factor, a is horizontal shift and b is vertical shift.

If 0<k<1, then the graph compressed vertically by factor k and if k>1, then the graph stretch vertically by factor k.

If a>0, then the graph shifts a units left and if a<0, then the graph shifts a units right.

If b>0, then the graph shifts b units up and if b<0, then the graph shifts b units down.

The given relationship between two function is

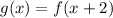`g(x)=f(x+2)` .... (2)

On comparing (1) and (2) we get

h=2> 0, so the graph of g(x) is the graph of ​f(x)​ translated 2 units left.

Therefore, the correct option is D.

Answer 2

Adding a value to the X value shifts the graph that many units to the left.

X+2 adds 2 to x, so the graph would shift 2 unites to the left.

The answer is:

The graph of g(x) is the graph of ​f(x)​ translated 2 units left.