Answer:
a. 4(d+3e)
b. 6(3x+5y)
c. 7(3a+4y)
d. 8(3f+7g)
Explanation:
In each case we find the greatest common factor of the numbers. That is the greatest number that goes into both the numbers. Then we factor it out in front and inside parentheses we divide each original term by the greatest common factor:
a. 4d+12e, GCF: 4

b. 18x+30y, GCF: 6
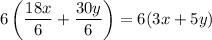
c. 21a+28y, GCF: 7

d. 24f+56g, GCF: 8
