Question

A person creates a game in which a person pays $5.00 to play. Then, they draw a single card from a standard

shuffled deck of cards.
If they draw a face card, they win $10.00 (i.e. they get their $5.00 back and get an extra $5.00).
If they draw an ace, they win $20.00 (i.e. they get their $5.00 back and get an extra $15.00).
With any other draw they lose their money.
What is the expected money value playing this game?
Allee sila
ITT:
SONTE
-$5.00
-$1.15
$1.31
$0.38

Answer 1

Explanation:

$5.00

Answer 2

Option B.

Explanation:

Cost of game = $5.00

If they draw a face card, they win $10.00 (i.e. they get their $5.00 back and get an extra $5.00).

Winning amount = $5

Number of face cards = 12

Probability of getting a face card =
`(12)/(52)=(3)/(13)`

If they draw an ace, they win $20.00 (i.e. they get their $5.00 back and get an extra $15.00).

Winning amount = $15

Number of ace = 4

Probability of getting an ace =
`(4)/(52)=(1)/(13)`

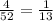
Any other draw they lose their money.

Number of other card (Exclude ace and face cards) = 52-4-12=36

Probability of getting other cards =
`(36)/(52)=(9)/(13)`

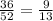
The expected money value playing this game is

E(x) = 5 × P(face cards) + 15 × P(Ace) - 5 × P(Other cards)

`E(x)=5* (3)/(13)+15* (1)/(13)-5* (9)/(13)`

`E(x)=(15)/(13)+(15)/(13)-(45)/(13)`

`E(x)=-(15)/(13)`

`E(x)=-1.15384615385`

`E(x)\approx -1.15`

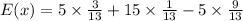
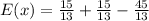
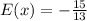
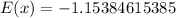
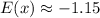
The expected money value playing this game is -$1.15

Therefore, the correct option is B.