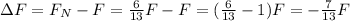Answer:
The new force
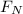 will be
will be
 times the old force F. The change then will be
times the old force F. The change then will be

Step-by-step explanation:
The force between two current-carrying parallel wires is calculated with the formula:
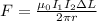
where r is the distance between them,
 a portion of length of the wires we consider,
a portion of length of the wires we consider,
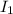 and
and
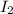 their current intensity and
their current intensity and
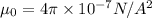 the vacuum permeability.
the vacuum permeability.
If the current in one wire is increased by a factor of 2, the current in the other is increased by a factor of 3 , and the distance between the wires is decreased by a factor of 13, then we would have a new situation N where (considering the previous variables as an initial situation):

And the force then will be:
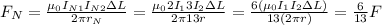
So the change will be: