Answer:
The probability of rolling a number greater than 4 or less than 3 is

Solution:
In the given question there are two events as follows:
(a) Rolling a number greater than 4 i.e. A = {5,6}
(b) Rolling a number less than 3 i.e. B = {1,2}
Since a die has 6 numbers,
P(A) =
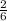 where P(A) is the probability of occurrence of event A and P(B) =
where P(A) is the probability of occurrence of event A and P(B) =
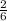
Since, Event A and Event B has nothing in common therefore they are mutually exclusive events.
P(A∪B) = P(A) + P(B)
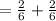
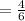
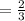
Therefore the probability of getting a number greater than 4 or less than 3 is
