Question

Many American home owners display U.S. flag in their front yard to show their patriotism. In a particular US state 60% of home owners are republicans and 40% are domocrats. A survey indicates that 40% of republicans and 20% of democrats home owners display flags. a. What is the probability that your neighbor is a republican? 5 pts. b. What is the probability that your neighbor is a democrat? 5 pts. c. You notice that your new neighbor hangs a U.S. flag. With this additional information, what is the probability that your neighbor is NOT a republican? 10 pts. d. You notice that your new neighbor hangs a U.S. flag. With this additional information, what is the probability that your neighbor is NOT a democrat? 10 pts.

Answer 1

Answer: a) 0.6, b) 0.4, c) 0.25, d) 0.75

Explanation:

Since we have given that

Republicans Domocrats Total

Display 0.24 0.08 0.32

Don't display 0.36 0.32 0.68

Total 0.6 0.4 1

a. What is the probability that your neighbor is a republican?

`P(R)=0.6`

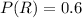
b. What is the probability that your neighbor is a democrat?

`P(D)=0.4`

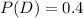
c. what is the probability that your neighbor is NOT a republican?

P(not a republican given that display flag) = 1 - P(republican given that display flag)

`=1-(P(R\cap F))/(P(F))\\\\=1-(0.24)/(0.32)\\\\=1-0.75\\\\=0.25`

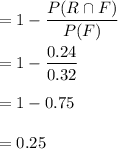
d) You notice that your new neighbor hangs a U.S. flag. With this additional information, what is the probability that your neighbor is NOT a democrat?

P(not democrat given that display flag) = 1 - P(democrat given that display flag)

`=1-(P(D\cap F))/(P(F))\\\\=1-(0.08)/(0.32)\\\\=1-0.25\\\\=0.75`

Hence, a) 0.6, b) 0.4, c) 0.25, d) 0.75