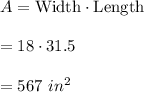Question

The sides of a rectangle are in the ratio 4:7. If its longer side is 31.5 in., find the shorter side, the perimeter, and the area of this rectangle.

Answer 1

Width = 18 inches

Perimeter = 99 inches

Area = 567 square inches

Explanation:

The sides of a rectangle are in the ratio 4:7. Then

Width
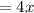`=4x` in

Length
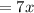`=7x` in.

If its longer side is 31.5 in, then

Length = 31.5 in

and

`7x=31.5\\ \\x=(31.5)/(7)=4.5\ in`

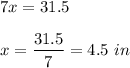
So,

Width
`=4\cdot 4.5=18\ in`

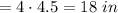
The perimeter is

`P=2(\text{Width}+\text{Length})\\ \\=2(18+31.5)\\ \\=2\cdot 49.5\\ \\=99\ in`

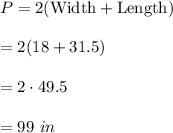
The area is

`A=\text{Width}\cdot \text{Length}\\ \\=18\cdot 31.5\\ \\=567\ in^2`