Answer:
The final temperature of the air is
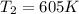
Step-by-step explanation:
We can start by doing an energy balance for the closed system
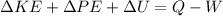
where
 = the change in kinetic energy.
= the change in kinetic energy.
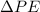 = the change in potential energy.
= the change in potential energy.
 = the total internal energy change in a system.
= the total internal energy change in a system.
Q = the heat transferred to the system.
W = the work done by the system.
We know that there are no changes in kinetic or potential energy, so
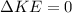 and
and
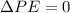
and our energy balance equation is

We also know that the paddle-wheel transfers energy to the air at a rate of 1 kW and the system receives energy by heat transfer at a rate of 0.5 kW, for 5 minutes.
We use this information to calculate the total internal energy change
 using the energy balance equation.
using the energy balance equation.
We convert the interval of time to seconds

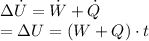
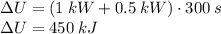
We can use the change in specific internal energy
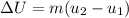 to find the final temperature of the air.
to find the final temperature of the air.
We are given that
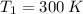 and the air can be describe by ideal gas model, so we can use the ideal gas tables for air to determine the initial specific internal energy
and the air can be describe by ideal gas model, so we can use the ideal gas tables for air to determine the initial specific internal energy
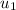

Next, we will calculate the final specific internal energy
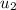
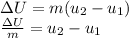

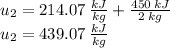
With the value
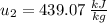 and the ideal gas tables for air we make a regression between the values
and the ideal gas tables for air we make a regression between the values
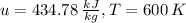 and
and
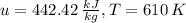 and we find that the final temperature
and we find that the final temperature
 is 605 K.
is 605 K.