Question

A rigid tank whose volume is unknown is divided into two parts by a partition. One side of the tank contains an ideal gas at 927°C. The other side is evacuated and has a volume twice the size of the part containing the gas. The partition is now removed and the gas expands to fill the entire tank. Heat is now transferred to the gas until the pressure equals the initial pressure. Determine the final temperature of the gas.

Answer 1

The final temperature will be 3600 K.

Step-by-step explanation:

Side 1

T=927°C

T=1200 K

Lets take volume of one side is V then the volume of other side will be 2V. The final volume of system will become 3 V.

The system is closed so the mass of system will remain constant .

We know that for ideal gas P V = m R T

The final pressure is same as initial pressure so we can say that

`(T_i)/(V_i)=(T_f)/(V_f)`

`(1200)/(V)=(T_f)/(2V+V)`

`T_f=3600\ K`

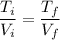
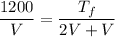
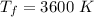
So the final temperature will be 3600 K.