Question

Provide an appropriate response. The mean score of a placement exam for entrance into a math class is 80, with a standard deviation of 10. Use the Empirical Rule to find the percentage of scores that lie between 60 and 80. (Assume the data set has a bell- shaped distribution.)

a.95%
b.34%
c.47.5%
d.68%

Answer 1

option (c) 47.5%

Step-by-step explanation:

Given:

Mean score into a math class, μ = 80

Standard deviation, σ = 10

Prescribed range between 60 and 80

Range in 1 standard deviation from mean

μ ± σ

i.e

80 - ( 1 × 10 ) and 80 + ( 1 × 10 )

or

70 and 90

our prescribed range of 60 and 80 does not lies in the obtained range

thus,

Range in 2 standard deviation from mean

μ ± σ

i.e

80 - ( 2 × 10 ) and 80 + ( 2 × 10 )

or

60 and 100

our prescribed range of 60 and 80 lies in the obtained range i.e within 2 standard deviations of the mean.

therefore,

The interval is half of that because it is the data between the mean and two standard deviations below the mean.

Hence,

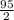`\frac{\textup{95}}{\textup{2}}` = 47.5%

Hence,

The correct answer is option (c) 47.5%