Answer:
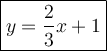
Explanation:
The slope-intercept form of an equation of a line:

m - slope
b - y-intercept → (0, b)
The fromual of a slope:
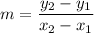
(x₁, y₁), (x₂, y₂) - points on a line
We have the points from the graph:
(0, 1) → b = 1 and (3, 3).
Calculate the slope:
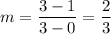
Finally we have the equation of a line:
