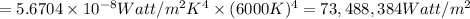Question

1) The Sun has an average surface temperature of approximately 6000 K. Calculate the power per unit area released by the surface of the Sun using Stefan-Boltzmann Law and report the value below. Units are a must. (2 points)

Power per unit area released by the Sun=

Answer 1

Power per unit area released by the Sun is 73,488,384 Watt per meter square.

Step-by-step explanation:

The Sun has an average surface temperature of approximately,T= 6000 K

The power per unit area released by the surface of the Sun be P'

Stefan-Boltzmann Law is given as:

`P'=(Power)/(Area)=\sigma T^4`

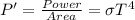
where

`\sigma =5.6704* 10^(-8) Watt/m^2K^4` Stefan-Boltzmann constant

T = Absolute temperature of the body

Power per unit area released by the Sun:

`=5.6704* 10^(-8) Watt/m^2K^4* (6000 K)^4=73,488,384 Watt/m^2`