Answer:
D.No, because some models of cell phones will have a larger market share than others. Measures from different models should be weighted according to their size in the population.
Explanation:
(a) Range is the difference between the smallest and largest observation.
Here Smallest observation = 0.63
and Largest observation = 1.48
⇒ Range = 0.85
(b) Standard Deviation is calculate by,
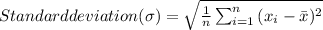
where,
 is mean of the observation.
is mean of the observation.
Here, Mean = 0.988
⇒ Standard Deviation = 0.313
(c) Variance is the square of Standard deviation.
Thus, Variance = (Standard Deviation)² = 0.098
(d) Here last option(D) is true i.e. No, because some models of cell phones will have a larger market share than others. Measures from different models should be weighted according to their size in the population.