Answer: 0.034
Explanation:
Given : P(Submitted under warranty)= 0.20
P(Replaced | Submitted under warranty)=0.40
P(Replaced and Submitted under warranty )= P(Submitted under warranty)×P(Replaced | Submitted under warranty)
=
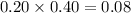
Let x be the number of telephones will end up being replaced under warranty.
Total telephones purchased : n= 10
Using binomial probability formula :

i.e. The probability that exactly three will end up being replaced under warranty will be :-
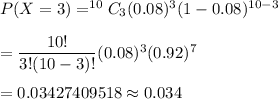 [Rounded to three decimal places. ]
[Rounded to three decimal places. ]
Hence, the probability that exactly three will end up being replaced under warranty : 0.034