Answer:
Speed, v = 7.83 m/s
Step-by-step explanation:
It is given that,
Length of the bridge, l = 5 m
The road on the far side is 2.0 m lower than the road on this side, x = 2 m
The horizontal distance covered by the car is 5 meters and the vertical distance covered by the car is 2 meters.
Initial speed of the car, u = 0
Let t is the time taken by the car . Using the second equation of motion as :
For vertical distance :


Let v is the velocity to jump the stream. It is given by :
Horizontal distance, d = 5 m
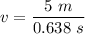
v = 7.83 m/s
So, the car should travel with a speed of 7.83 m/s. Hence, this is the required solution.