Question

A roller coaster has a "hump" and a "loop" for riders to enjoy (see picture). The top of the hump has a radius of curvature of 12 m and the loop has a radius of curvature of 15 m. (a) When going over the hump, the coaster is traveling with a speed of 9.0 m/s. A 100-kg rider is traveling on the coaster. What is the normal force of the rider’s seat on the rider when he is at the peak of the hump? Compare this with the normal force he would experience when the coaster is at rest. (b) What is the minimum speed the coaster must have at the top of the loop in order for the rider to remain in contact with his seat? Is this speed dependent on the mass of the rider? 8.

Answer 1

Part a)

`F_n = 306 N`

Part B)

`v = 12.1 m/s`

Step-by-step explanation:

Part A)

At the top of the hump the force on the rider is

1) Normal force

2) weight

so here we know that

`mg - F_n = (mv^2)/(R)`

`F_n = mg - (mv^2)/(R)`

`F_n = (100)(9.81) - (100(9^2))/(12)`

`F_n = 306 N`

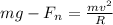
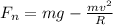
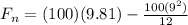
Part B)

At the top of the loop we will have

`F_n + mg = (mv^2)/(R)`

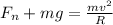
in order to remain in contact the normal force must be just greater than zero

so we will have

`mg = (mv^2)/(R)`

`v = √(Rg)`

`v = √(15* 9.81)`

`v = 12.1 m/s`