Question

A baseball is thrown horizontally from a height of 9.60 m above the ground with a speed of 30.0 m/s. Where is the ball after 1.40 s have clapsed?

Answer 1

The baseball will be 42 meters horizontally from where it was thrown and at the heigth of the ground.

Step-by-step explanation:

this is a problem that involves basic mechanics in two directions, for the x axis we have a constant speed problem and for the y axis we have a constant aceleration problem, using the equations for each one of these we got:

`X=Xo+Vox*t`

`Y=Yo+Voy*t+(a*t^(2) )/(2)`

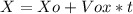
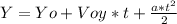
where Xo and Yo are the inital position for x and y axis, in this case
`Xo=0` and
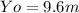`Yo =9.6m`, Vox and Voy are the initial speed for x and y axis, in this case
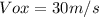`Vox=30m/s` and
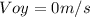`Voy=0m/s` since the ball was throwns horizontally, and a is the acceleration in the y axis which is the gravitational aceleration, that means
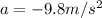`a=-9.8m/s^(2)`(the minus sign is because the gravitational aceleration is towards the negative y axis).

Replacing these values we have to equations that will help us find the position of the ball at any time we want:

`X=0+30m/s*t`

`Y=9.6m+0*t-(9.8m/s^(2)*t^(2))/(2)=9.6m-(9.8m/s^(2)*t^(2) )/(2)`

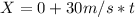
and here we just have to replace the time value and solve, so:

`X=0+30m/s*1.4s=42m`

`Y=9.6m-(9.8m/s^(2)*1.4s^(2) )/(2)=9.6m-4.9m/s^(2)*1.96s^(2)=9.6m-9.6m=0m`

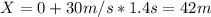
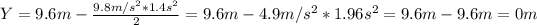
and that's it, the ball is 42 meters horizontally from where it was throwns and at the heigth of the ground.