Question

A lamp has a current of 2.17 A. In hours, how long does it take for 1 mole of electrons to pass through the lamp?

Answer 1

Answer: The amount of time needed for 1 mole of electrons to pass through the lamp is 12.33 hrs.

Step-by-step explanation:

We are given:

Moles of electron = 1 mole

According to mole concept:

1 mole of an atom contains
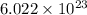`6.022* 10^(23)` number of particles.

We know that:

Charge on 1 electron =
`1.6* 10^(-19)C`

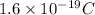
Charge on 1 mole of electrons =
`1.6* 10^(-19)* 6.022* 10^(23)=9.6352* 10^4C`

To calculate the time required, we use the equation:

`I=(q)/(t)`

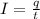
where,

I = current passed = 2.17 A

q = total charge =
`9.6352* 10^4C`

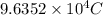
t = time required = ?

Putting values in above equation, we get:

`2.17A=(9.6352* 10^4C)/(t)\\\\t=(9.6352* 10^4C)/(2.17A)=44402s`

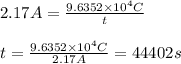
Converting this into hours, we use the conversion factor:

1 hr = 3600 seconds

So,
`44402s* (1hr)/(3600s)=12.33hr`

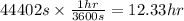
Hence, the amount of time needed for 1 mole of electrons to pass through the lamp is 12.33 hrs.