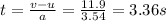Question

A world-class sprinter can reach a top speed (of about 11.9 m/s ) in the first 20.0 m of a race. What is the average acceleration of this sprinter?

Express your answer with the appropriate units.
How long does it take her to reach that speed?
Express your answer with the appropriate units.

Answer 1

1)
`3.54 m/s^2`

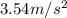
The average acceleration of the sprinter can be found by using the following SUVAT equation:

`v^2-u^2=2ad`

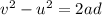
where

v is the final velocity

u is the initial velocity

a is the acceleration

d is the distance covered

In this problem,

u = 0 (the sprinter starts from rest)

v = 11.9 m/s

d = 20.0 m

Solving for a, we find the acceleration:

`a=(v^2-u^2)/(2d)=((11.9)^2)/(2(20))=3.54 m/s^2`

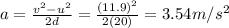
2) 3.36 s

We can find the time needed to reach this speed by using the SUVAT equation:

`v=u+at`

where

v is the final velocity

u is the initial velocity

a is the acceleration

t is the time

Here we have

u = 0

v = 11.9 m/s

a = 3.54 m/s^2

Solving for t, we find the time:

`t=(v-u)/(a)=(11.9)/(3.54)=3.36 s`