Answer:
661284 lb-ft
Step-by-step explanation:
We are given that height of water tank=18 ft
Radius of circular tank=12 ft
Density of water=
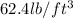

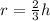
We have to find the work done in pumping all of the water over the top of the tank.
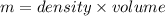
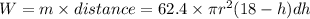
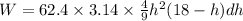
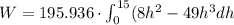
![W=195.936* [(8h^3)/(3)-(h^4)/(9)]^(15)_0](https://img.qammunity.org/2020/formulas/physics/high-school/o14m8kyl718a9ue3jk27jjtixjn2paen8c.png)
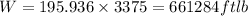
Hence, the work done in pumping all of the water over the top of the tank=661284 ft lb.