Answer:
a. Mean = 72.35
Standard deviation = 16.68
Mean =

and,
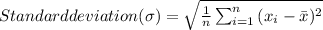
where,
 is mean of the distribution.
is mean of the distribution.
b. The passing grade is 75.
The students whose grade is less than 75 are fail
There are 10 students whose grade are more than 75.
Thus, Probability of pass students = 10 ÷ 20 = 0.5
c. There are 10 students that score between 75 and 95.
∴ Probability = 10 ÷ 20 = 0.5
d. The 15% of 20 = 3 students.
Thus the score will put a student in the bottom 15% = 48
e. Thus, the score will put a student in the top 2% = 95