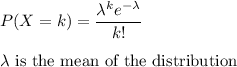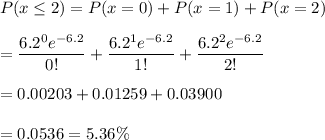Answer:
1) Poisson distribution with mean 6.2 and standard deviation 2.49.
2) 0.0536
Explanation:
We are given the following information in the question:
Mean of daily surgeries = 6.2
a) The count of daily surgeries can be treated as a Poisson distribution.
- The Poisson distribution is the discrete probability distribution of the number of events occurring in a given time period, given the average number of times the event occurs over that time period.
- The variance of Poisson distribution is equal to the mean of Poisson distribution.
Variance = 6.2
Standard deviation =
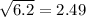
a) Poisson distribution with mean 6.2 and standard deviation 2.49.
2) P( 2 or less surgeries)
Formula: