Answer and explanation:
Given : Assume that each of your calls to a popular radio station has a probability of 0.02 of connecting, that is, of not obtaining a busy signal. Assume that your calls are independent.
This form an geometric probability,
Probability that call connects p=0.02
Probability that call does not connect q=1-0.02=0.98
To find :
(a) What is the probability that your first call that connects is your 10th call?
The formula is given by,
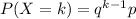
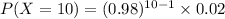


(b) What is the probability that it requires more than five calls for you to connect?


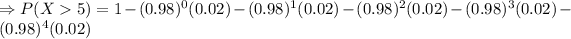
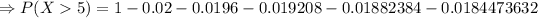
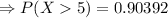
(c) What is the mean number of calls needed to connect?
The mean value of a geometric distribution with probability of success = p is given by -
![\mu = E[X] = (1)/(p)](https://img.qammunity.org/2020/formulas/mathematics/college/nonoi1sxzupit12efz8pp67u800rzby44a.png)
![\mu = E[X] = (1)/(0.02)](https://img.qammunity.org/2020/formulas/mathematics/college/b4vnb97amhzf0ihwauidkaip61vkly9zpb.png)
![\mu = E[X] =50](https://img.qammunity.org/2020/formulas/mathematics/college/m1g4fdimniejcxn3lhtmipx7uz2a2ytsli.png)