Question

Determine algebraically if the following equations represent parallel or perpendicular lines and justify your answer. : -2x+5y=-10 and -4y-10x=12

Answer 1

The given equations -2x+5y=-10 and -4y-10x=12 are perpendicular lines.

Solution:

From question,given equations are -2x+5y=-10 and -4y-10x=12

Two equations or lines are perpendicular only if they are negative reciprocals of each other.

To find the slope of an equation we write the equation in the slope intercept form which given as,

y = mx+C

where “m” is the slope of the line and “C” is the y-intercept.

Step 1:

Writing equation -2x+5y=-10 in slope intercept form

-2x+5y=-10

Shifting -2x to the right hand side

5y = 2x -10

By dividing both sides of the equation by “5”, we get

`\mathrm{y}=\frac{2 \mathrm{x}-10}{5}`

`\mathrm{y}=(2)/(5) \mathrm{x}-(10)/(5)`

`\mathrm{y}=(2)/(5) \mathrm{x}-2`

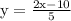
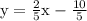
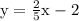
By comparing the above equation with y = mx+C, we get the value of “m” =
`(2)/(5)`

Hence slope of the equation -2x+5y=-10 is m =
`(2)/(5)`

Step 2:

Writing equation -4y-10x =12 in slope intercept form

-4y-10x =12

Shifting -10x to the right hand side in above equation, we get

-4y = 10x+12

By dividing both sides of the equation by “-4”, we get

`\mathrm{y}=\frac{10 \mathrm{x}+12}{-4}`

`\mathrm{y}=(10)/(-4) \mathrm{x}+(12)/(-4)`

`y=-(5)/(2) x-3`

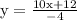
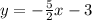
By comparing the above equation with y = mx+C , we get the value of “m” as
`(-5)/(2)`

Hence slope of the equation -4y-10x =12 is m=
`(-5)/(2)`

The slope of the equation -4y-10x =12, m =
`(-5)/(2)` is negative reciprocal of the slope of the equation -2x+5y=-10, m =
`(2)/(5)`, thus the equations are perpendicular lines.