Question

On July 1, 1990, John invested $300 in an account that earned 8% simple interest. On July 1, 1993 he closed this account and deposited the liquidated funds in a new account earning q% simple discount. On July 1, 1998, John had a balance of $520 in the simple discount account. How much interest did he earn between July 1, 1993 and July 1, 1994?

Answer 1

q =5.7% $21.204

Step-by-step explanation:

The simple interest formula is

I =
`(P*R*T)/(100)`

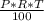
Given that

I = Interest , T = time ; ;R is rate ; P = principal

The amount of Interest that John earned by July 1, 1993 is,

I =
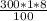`(300*1* 8)/(100)` = 72

Thus, the total amount in the account by July 1 1993

= $300 + $72= $372

We understand that he deposited this amount to earn interest at q rate

On July 1, 1998, John received $520. This means that his interest in the five years = $520-$372 = $148

Using the simple interest formula,

Interest = PRT/100

148 =
`(520*5*q )/(100)`

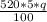
= 14,800 =2600q

q =
`(14,800)/(2,600)`

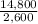
q = 5.69231%

Thus, rate = 5.7%

The interest that John earned between July 1, 1993 and July 1,1994 can be worked as

I =
`(PRT)/(100) = (372*5.7*1)/(100)`

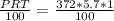
= $21.204