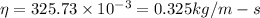Question

Stokes' law describes sedimentation of particles in liquids and can be used to measure viscosity. Particles in liquids achieve terminal velocity quickly. One can measure the time it takes for a particle to fall a certain distance and then use Stokes' law to calculate the viscosity of the liquid. Suppose a steel ball bearing (density 7.8 ✕ 103 kg/m3, diameter 2.4 mm) is dropped in a container of motor oil. It takes 10 s to fall a distance of 0.75 m. Calculate the viscosity (in kg/(m·s)) of the oil.

Answer 1

Answer:
`\eta =325.73* 10^(-3)=0.325 kg/m-s`

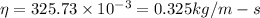
Step-by-step explanation:

Given

density
`(\rho )=7.8* 10^3 kg/m^3`

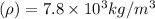
Diameter(d)=2.4 mm

time taken=10 s

Distance moved(h)=0.75 m

At terminal velocity Drag force is equal to Weight

`F_D=mg`

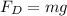
Volume of ball
`=(4\pi r^3)/(3)=7.23 mm^3`

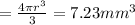
Mass of ball
`=\rho v=7.23* 7.8* 10^3* 10^(-9)=56.39* 10^(-6) kg`

`F_D=56.39* 10^(-6)* 9.8=552.66* 10^(-6) N`

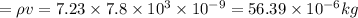
Also for spherical bodies drag force is equal to Stock Force

`F_s=6* \pi * \eta * r* v_r`

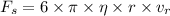
Where
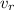`v_r`= Terminal velocity

`v=(h)/(t)=(0.75)/(10)=0.075 m/s`

`552.66* 10^(-6)=6* pi* \eta * \1.2* 10^(-3)* 0.075`

`\eta =325.73* 10^(-3)=0.325 kg/m-s`