Question

5. During the eruption of Mount St. Helens in 1980. debris was ejected at a speed of over 440 feet per second

(300 miles per hour). The elevation in feet above sea level of a rock ejected at an angle of 75° above

horizontal is given by the function y(1) --1612 +425t+8200 where t is the time in seconds after the

iption. The rock's horizontal distance in feet from the point of ejection is given by x(I) = 1131. If the rock

Landed at an elevation of 6000 feet, what is the horizontal distance from the point of ejection to where it!

landed rounded to the nearest hundred feet?

Answer 1

3500 ft

Explanation:

We assume you intend the equations to be ...

`y(t)=-16t^2+425t+8200\\x(t)=113t`

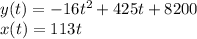
Then we can solve the second equation for t and use that in the first equation. We can solve the first equation for y=6000.

x = 113t

t = x/113

y(t) = y(x/113) = -16(x/113)² +425(x/113) +8200 = 6000

(16/12769)x² -(425/113)x -2200 = 0 . . . . . . subtract 6000, multiply by -1

Using the quadratic formula, the positive solution is ...

x = ((425/113) +√((-425/113)² -4(16/12769)(-2200))/(2(16/12769))

The fraction in the denominator can be inverted and used as a multiplier. We also use a√b = √(a²b) to simplify the radical.

x = (48025 +√4104275825)/32

x ≈ 3502.8

Rock was thrown about 3500 feet horizontally.