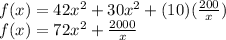Question

A rectangular box is to have a square base and a volume of 50 ft3. The material for the base costs 42¢/ft2, the material for the sides costs 10th¢/ft2, and the material for the top costs 30¢/ft2. Letting x denote the length of one side of the base, find a function in the variable x giving the cost (in dollars) of constructing the box.

Answer 1

`f(x)=72x^(2) +(2000)/(x)`

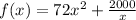
Explanation:

1) First of all, we are going to see what is the area of each element of the box.

The area of the base is x² (because x is the length of the side of the square base).

The area of the top is x² as well.

Now, the area of each side will be x·h where h is the height of the box. The box has 4 sides so the total area of the sides will be 4x·h

However, we can express h in terms of x because we have the total volume of the box:

V = (base area) · height = 50 ft³

50 = x²h

50/x² = h

Therefore, the area of the 4 sides will be:
`4(x)(h) = 4x((50)/(x^(2) ) )=(200)/(x)`

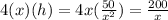
2) Now we are going to find the function giving the cost of constructing the box:

To find the function, we are going to use the prices we are given.

The price of the base, top and sides will be (for each of them): (price per ft²)(area in ft²)

Therefore the function to find the price (in cents) would be:

`f(x)=42x^(2) +30x^(2) +(10)((200)/(x) )\\f(x)=72x^(2) +(2000)/(x)`