Question

Find the center, vertices, and foci of the ellipse with equation 4x^2 + 9y^2 =36

Answer 1

Center: (0, 0); Vertices: (-3, 0), (3, 0); Foci: (-
`√(5)`,0), (
`√(5)`,0)

Explanation:

Answer 2

The center, vertices and foci of the ellipse with equation
`4 x^(2)+9 y^(2)=36 is (0,0),(\pm 3,0),(\pm √(5), 0)` respectively

Solution:

The equation of ellipse with centre (0, 0) in the form of
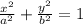`(x^(2))/(a^(2))+(y^(2))/(b^(2))=1` --- eqn 1

Where,

x is the major axis

Centre (0, 0)

Vertices is
`(\pm \mathrm{a}, 0)`

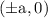
Foci is
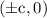`(\pm \mathrm{c}, 0)` where
`c=\sqrt{a^(2)-b^(2)}`

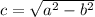
Now given that the equation of ellipse is

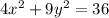`4 x^(2)+9 y^(2)=36` --- eqn 2

On dividing equation (2) by 36,

`(x^(2))/(9)+(y^(2))/(4)=1`

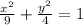
On comparing equations (1) and (2),

We get a = 3, b= 2

`c=\sqrt{a^(2)-b^(2)}=√(9-4)=√(5)`

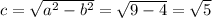
So centre of
`(x^(2))/(9)+(y^(2))/(4)=1 is (0, 0)`

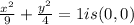
Vertices of
`(x^(2))/(9)+(y^(2))/(4)=1 is (\pm 3,0)`

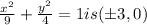
Foci of
`(x^(2))/(9)+(y^(2))/(4)=1 is (\pm √(5), 0)`