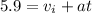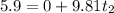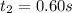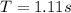Answer:
Part a)
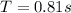
Part b)
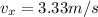
Part c)
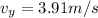
Part d)

Part e)
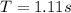
Step-by-step explanation:
Part a)
initial vertical position = 1.02 m
maximum height = 1.80 m
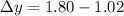
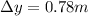
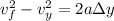

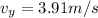
time taken by it to reach this height
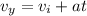
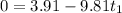
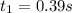
Now when it again touch the ground then its speed is given as
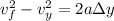
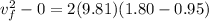
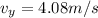
time taken by it to reach this height
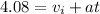
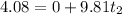
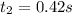
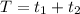
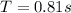
Part b)
Horizontal velocity
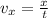
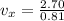
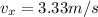
Part c)
vertical velocity is the intial y direction velocity
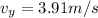
Part d)
Take off angle is given as
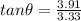

Part e)
initial vertical position = 1.20 m
maximum height = 2.50 m
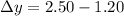
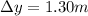
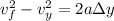
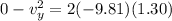
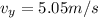
time taken by it to reach this height
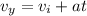
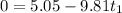
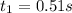
Now when it again touch the ground then its speed is given as
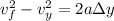
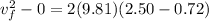
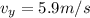
time taken by it to reach this height