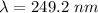Answer:
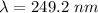
Step-by-step explanation:
Given that:
The work function of the rhodium = 480.5 kJ/mol
It means that
1 mole of electrons can be removed by applying of 480.5 kJ of energy.
Also,
1 mole =

So,
 electrons can be removed by applying of 480.5 kJ of energy.
electrons can be removed by applying of 480.5 kJ of energy.
1 electron can be removed by applying of
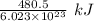 of energy.
of energy.
Energy required =

Also,
1 kJ = 1000 J
So,
Energy required =

Also,
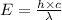
Where,
h is Plank's constant having value
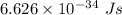
c is the speed of light having value
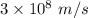
So,
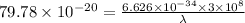

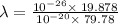
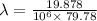
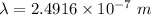
Also,
1 m = 10⁻⁹ nm
So,