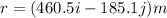Question is missing. Found on google:
a) What are the components of the acceleration of the fish?
(b) What is the direction of its acceleration with respect to unit vector î?
(c) If the fish maintains constant acceleration, where is it at t = 30.0 s?
(a)
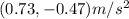
The initial velocity of the fish is
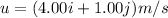
while the final velocity is

Initial and final velocity are related by the following suvat equation:

where
a is the acceleration
t is the time
The time in this case is t = 15.0 s, so we can use the previous equation to find the acceleration, separating the components:
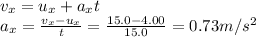
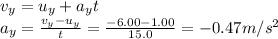
(b)
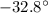
The direction of the acceleration vector with respect to i can be found by using the formula
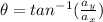
where
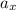 is the horizontal component of the acceleration
is the horizontal component of the acceleration
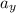 is the vertical component of the acceleration
is the vertical component of the acceleration
From part a), we have
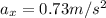
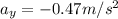
Substituting,
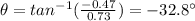
(c)
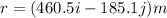
The initial position of the fish is

The generic position r at time t is given by

where
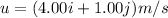 is the initial velocity
is the initial velocity
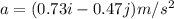 is the acceleration
is the acceleration
Substituting t = 30.0 s, we find the final position of the fish. Separating each component:
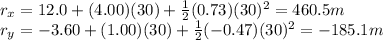
So the final position is