Answer:
Option B) 18
Explanation:
We are given the following information in the question:
Ratio of employees with advanced degrees to employees with bachelor degrees = 10:25
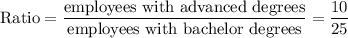
Then, we can write:
Total number of employees with advanced degrees = 10x
Total number of employees with bachelor degrees = 25 x
Total number of employees =
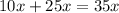
Total number of employees = 63
We are given that:
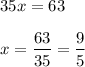
Number of employees with advanced degree =
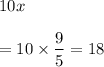
Thus, there are 18 employees with advanced degree.
Option B) is the correct answer.