Answer:
The second ball traveled more distance, horizontally and vertically.
Step-by-step explanation:
The given function is
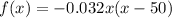
To find the distances that the second football travels, we need to find the vertex of its movement, because it's movement has a parabola form. The quadratic expression is

Where
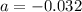 and
and
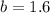
The vertex has coordinates of
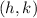 , where
, where

Replacing values, we have
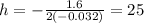
Then,
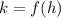
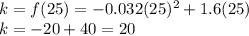
Which means the maximum height of the second football is 20 yards. That means it travels 40 yards vertically.
Now, its horizontal distance can be found when
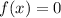
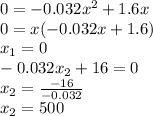
So, its horizontal distance is 500 yards.
Comparing the distances between the footballs.
Ball 1
Horizontal distance of 30 yards.
Vertical distance of 30 yards.
Ball 2
Horizontal distance of 500 yards.
Vertical distance of 40 yards.
If we find their difference, it would be
Horizontal: 500 - 30 = 470 yards.
Vertical: 40 - 30 = 10 yards.
Therefore, the second ball traveled more distance, horizontally and vertically.