Question

Urea (H2NCONH2) is used extensively as a nitrogen source in fertilizers. It is produced commercially from the reaction of ammonia and carbon dioxide.

2 NH3(g) + CO2(g) reaction arrow identifying the need for heat and pressure to be applied H2NCONH2(s) + H2O(g)

Ammonia gas at 223°C and 90. atm flows into a reactor at a rate of 580. L/min. Carbon dioxide at 223°C and 48 atm flows into the reactor at a rate of 600. L/min. What mass of urea is produced per minute by this reaction assuming 100% yield?

Answer 1

38,456.4 grams of urea is produced per minute by this reaction.

Step-by-step explanation:

`2 NH_3(g) + CO_2(g)\rightarrow H_2NCONH_2(s) + H_2O(g)`

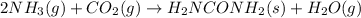
Ammonia gas at 223°C and 90 atm flows into a reactor at a rate of 580 L/min.

Volume of ammonia = V = 580 L/min

Temperature of the gas = T = 223°C = 496 K

Pressure of the ammonia gas = P = 90 atm

Moles of ammonia per minute = n

`PV=nRT`

`n=(90 atm* 580 L/min)/(0.0821 atm L/mol K* 496 K)`

`n=1,281.87 mol/min`

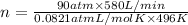
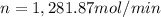
Carbon dioxide at 223°C and 48 atm flows into the reactor at a rate of 600 L/min.

Volume of gas= V = 600 L/min

Temperature of the gas = T = 223°C = 496 K

Pressure of the ammonia gas = P = 48 atm

Moles of ammonia per minute = n

`PV=nRT`

`n=(48 atm* 600 L/min)/(0.0821 atm L/mol K* 496 K)`

`n=707.24 mol/min`

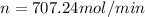
Moles of ammonia gas per minute = 1,281.87 mol

Moles of carbon dioxide per minute = 707.24 mol

According to reaction 2 mol of ammonia gas reacts with 1 mol of carbon dioxide.

Then 1,281.87 moles of ammonia will react with:

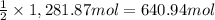`(1)/(2)* 1,281.87 mol=640.94 mol` of carbon dioxide.

Carbon dioxide is in excess amount. Amount of urea will depend upon amount of ammonia.

According to reaction 2 mol of ammonia gas gives 1 mol of urea.

Then 1,281.87 moles of ammonia will give:

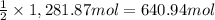`(1)/(2)* 1,281.87 mol=640.94 mol` of urea.

Mass of 640.94 moles of urea : 640.94 mol × 60 g/mol=38,456.4 g

38,456.4 grams of urea is produced per minute by this reaction.

Answer 2

The mass of urea produced is 2.175kg/min

Step-by-step explanation:

Step 1: The balanced equation

2 NH3(g) + CO2(g) → H2NCONH2(s) + H2O(g)

Step 2: Given data

Ammonia gas (NH3) at 223°C (= and 90 atm flows into a reactor at a rate of 580 L/min

Carbon dioxide( CO2) at 223°C and 48 atm flows into the reactor at a rate of 600. L/min.

Step 3: Calculate

⇒NH3:

(P1*V1)/ T1 = (P2*V2)/T2

P1 = 90 atm

V1 = 670L

T1 = 496 K

P2 = 1 atm

V2 = TO BE DETERMINED

T2= 273K

P1V1T2 = P2V2T1

90atm*670L*273K = 1atm*V2*496K

V2 = (90 x 670 x 273)/496

V2 = 33189 L ( Volume at STP)

At STP 1 mole = 22.4 L

This means 33,189/22.4 = 1,482 moles

This gives us a rate of 1482g /min NH3

CO2:

P1 = 47 atm

V1 = 600L

T1 = 496 K

P2 = 1 atm

V2 = TO BE DETERMINED

T2= 273K

47atm*600L*273K = 1atm*V2*496K

V2 = (47*600*273) /496

V2 = 15,521L

15,521 ÷ 22.4 = 692.92 moles = 692.92g/min

1482g /min NH3 + 692.92g/min = 2.175kg/min