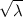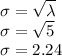Question

In a typical children's soccer game, there is one goal scored approximately every 18 minutes. The number of goals scored follows a Poisson distribution. What would the standard deviation be for the number of goals scored in an entire game? (A game consists of two 45-minute halves.)

Answer 1

2.24

Explanation:

The probability formula using a Poisson distribution is:

`P(k\ events) = (\lambda^(k)e^(-\lambda))/(k!) \\\lambda\ is\ the\ average\ number\ of\ events\ per\ interval \\e\ is\ euler's\ number \\k\ is\ the\ number\ of\ events\ you\ want\ to\ calculate`

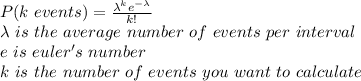
λ = 90 / 18 = 5 average goals per interval (interval = a game)

So if for example you were interested in the probability of making 2 goals in a game

k = 2

`P(k = 2) = (5^(2)e^(-5))/(2!) = 0.084`

This was just an example,

The standard deviation is
`√(\lambda)`

`\sigma = √(\lambda) \\\sigma = √(5) \\\sigma = 2.24`